Subpage of Calorimetry of the PD-D2O System: from Simplicity via Complications to Simplicity.
The purpose of this subpage is to study the section named below. Comments here should be aimed toward study and learning as to what is in the Original paper. This is not a place to argue “right” and “wrong,” but to seek agreement, where possible, or to delineate unresolved issues. General comments may be made on the Open discussion subpage.
General Features of our Calorimetry
Our approach to the measurement of excess enthalpy generation in Pd and Pd-alloy
cathodes polarised in D2O solutions has been described in detail elsewhere (see especially (1-5); see also (6)). The form of the calorimeter which we currently use is illustrated in Fig 1. The following features are of particular importance:
(i) at low to intermediate temperatures (say 20-50°C) heat transfer from the cell is dominated by
radiation across the vacuum gap of the lower, unsilvered, portion of the Dewar vessel to the
surrounding water bath (at a cell current of 0.5A and atmospheric pressure of 1 bar, the cooling due to evaporation of D2O reaches 10% of that due to radiation at typically 95-98°C for Dewar cells of the design shown in Fig 1).
(ii) the values of the heat transfer coefficients determined in a variety of ways (see below) both with and without the calibrating resistance heater (see Fig 2 for an example of the temperature-time and cell potential-time transients) are close to those given by the product of the Stefan-Boltzmann coefficient and the radiant surface areas of the cells.
(iii) the variations of the heat transfer coefficients with time (due to the progressive fall of the level of the electrolyte) may be neglected at the first level of approximation (heat balances to within 99%) as long as the liquid level remains in the upper, silvered portions of the calorimeters.
(iv) the room temperature is controlled and set equal to that of the water baths which contain
secondary cooling circuits; this allows precise operation of the calorimeters at low to intermediate
temperatures (thermal balances can be made to within 99.9% if this is required).
(v) heat transfer from the cells becomes dominated by evaporation of D2O as the cells are driven to the boiling point.
(vi) the current efficiencies for the electrolysis of D2O (or H2O) are close to 100%.
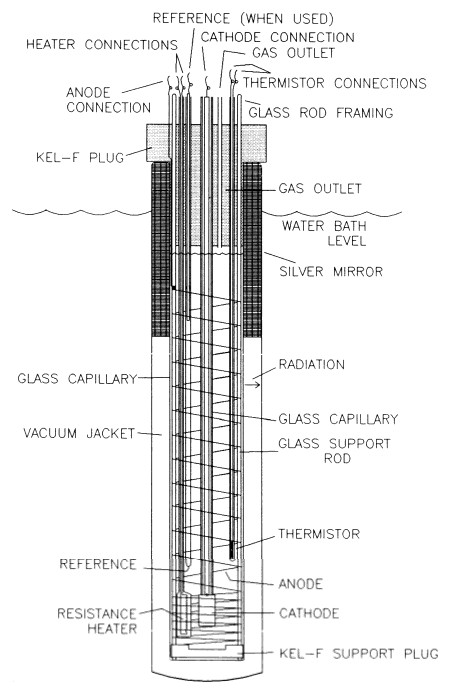
Figure 1. Schematic diagram of the single compartment open vacuum Dewar calorimeter cells used in this work.
Figure 2. Segment of a temperature-time/cell potential-time response (with 0.250 W heat calibration pulses) for a cell containing a 12.5 × 1.5mm platinum electrode polarised in 0.IM LiOD at 0.250A.
References (for this section)
1. Martin Fleischmann, Stanley Pons, Mark W. Anderson, Liang Jun Li and Marvin
Hawkins, J. Electroanal. Chem., 287 (1990) 293. [copy]
2. Martin Fleischmann and Stanley Pons, Fusion Technology, 17 (1990) 669. [Britz Pons1990]
3. Stanley Pons and Martin Fleischmann, Proceedings of the First Annual Conference on Cold Fusion, Salt Lake City, Utah, U.S.A. (28-31 March, 1990). [unavailable]
4. Stanley Pons and Martin Fleischmann in T . Bressani, E. Del Guidice and G.
Preparata (Eds), The Science of Cold Fusion: Proceedings of the II Annual Conference on Cold Fusion, Como, Italy, (29 June-4 July 1991), Vol. 33 of the Conference Proceedings, The Italian Physical Society, Bologna, (1992) 349, ISBN 887794-045-X. [unavailable]
5. M. Fleischmann and S. Pons, J. Electroanal. Chem., 332 (1992) 33. [Britz Flei1992]
6. W. Hansen, Report to the Utah State Fusion Energy Council on the Analysis of Selected Pons-Fleischmann Calorimetric Data, in T. Bressani, E. Del Guidice and G. Preparata (Eds), The Science of Cold Fusion: Proceedings of the II Annual Conference on Cold Fusion, Como, Italy, (29 June-4 July 1991), Vol. 33 of the Conference Proceedings, The Italian Physical Society, Bologna, (1992) 491, ISBN 887794-045-X. [link]


Some comments on this section.
While these comments all seem understandable and plausible i have two caveats:
(1) What is true in general might not be true in some corner case. Saying these are general features is not the same as saying that they are guaranteed to apply all of the time.
(2) As a statement that heat transfer coefficients remain the same:
(iii) the variations of the heat transfer coefficients with time (due to the progressive fall of the level of the electrolyte) may be neglected at the first level of approximation (heat balances to within 99%) as long as the liquid level remains in the upper, silvered portions of the calorimeters.
Contains a significant qualification. It deals only with variations due to progressive fall of the electrolyte under this condition (that the liquid remains in the upper regions). It leaves open the possibility of other variations from different causes during this regime.
It will be worth tracking whether in subsequent work there is an implicit assumption that there are no such other variations.
Thanks.
Are there any other plausible and significant causes for variation in the heat transfer coefficient? The cell design leads to heat transfer being almost entirely by radiation across the vacuum of the Dewar, in the unsilvered region. The silvered region will not allow transfer. When the liquid level falls below that, then heat transfer rate may decline as the liquid level falls. (I’m not sure. Heat transfer is from the inner wall of the Dewar to the outer wall, and that is constant. However, the inner wall may not be uniform in temperature where it is not in contact with the liquid. How well does the glass conduct heat?)
As long as the liquid level is complete in the lower part of the Dewar, the surface area allowed to radiate would be constant. The emissivity of the liquid should remain approximately the same.
(for others, a Dewar is like a Thermos bottle, it is very well insulated, but it is only partially a Thermos, the lower part is merely a vacuum bottle with no silvering as in a Thermos.)
Notice they don’t say they will remain the same, but they write about the obvious possible variation, and they are only talking about the “first level of approximation,” where the system “balances” within 99% (which I think refers to heat measured from calibration pulses.)
I think you agree with “general features,” with the caveat you gave.
heat loss through the top of the cell (Kel-F plug and associated through connections) will depend on cell conditions and this (as in CCS/ATER or CCS -something else TBC) could make significant changes.
Also worth nothing the cell diagram. For this cell the vapour exit is narrow, through the Kel-F which might cool outgassing vapour condensing steam. Also any turbulence in the cell could result in water entering this tube and being pushed out of the cell. Obviously these issues can be checked, but in absence of explicit checking it is worth noting this potential (variable based on cell conditions) issue in the calorimetry.
You are bringing up classical skeptical commentary. What is in the article about heat loss other than from the lower cell body into the cooling bath? The vapor exit is a narrow tube, to make entry of liquid into the tube unlikely. These are issue addressed in calibrations, I’d think. We will list possible unchecked conditions, and see if it is possible to quantify them. The major turbulence under boil-off conditions would be in a cell that, by then, has relatively little liquid in it, making escape unlikely, but … these are all items to be noted. Whatever issues have not been resolved will be presented to experts. Previously, I consulted Dieter Britz, as an example. I will probably consult with Miles, who may know more about FP calorimetry than anyone else alive, setting aside Pons himself, perhaps. When we have a list of questions, we can then search for answers.
The Kel-F top is not in good thermal contact with the electrolyte inside the cell (only with the gas phase). It is not in good thermal contact with the constant-temperature bath. However, it is in contact with air. How good an insulator is it? If it cools the gas escape tube, yes, water will condense on the inside, but that water may be considered as having been vaporized (if there is significant flow, this is a capillary tube, if I’m correct, and significant condensed water would readily be blown out. It would not be carrying the salt.)
Biberian knows a great deal about this calorimeter.
When we have more meat here, I will notify him and invite comment, though anyone can do that before me, I’d prefer, however, he be presented with better coverage and more focused questions. The same with Miles.
My method here is to raise issues I’m not sure about that are not settled in the paper. Neither me nor you can simply resolve them, so we end up with a list. Many get resolved by the paper, some perhaps can be resolved by expert analysis though care is needed, as well as expertise.
Documenting all this is useful.
As you have noted we have a putative mechanism for liquid-phase water loss without salt loss. Whether it could be significant later on – we do not yet know.
The mechanism described is actually a loss of evaporated water, not liquid phase. “Loss”
from what? From the calorimeter, which is the region of the cell with a defined and stable heat flow path into the surrounding water bath. Whatever enters the capillary would affect heat in the calorimetric region according to how it enters that tube. If it enters as a liquid, it would have salt content, which is suggested would show up as deposits outside. This would be “entrained water.”
If it enters as vapor, it has been evaporated in the cell and this is a loss of much more heat. That this heat ends up in the cap, perhaps, is, if relevant at all, only marginally relevant. If I’m correct, that capillary would rapidly heat to the boiling point and condensation would decline. Only if the condensed pure water were to fall back into the cell could this affect the heat calculations. Because it’s a capillary, and under boiling conditions in the cell, this water would not be expected to fall back, because vapor flow would carry it out.
What are the dimensions of the various objects here? The capillary tube? The Kel-F cap? What is the heat conductivity of Kel-F?
What remains for more detailed consideration is entrained water, not water condensation from vapor in the gas escape path.
Thanks for thinking of all possibilities. We want to be thorough here.